How To Draw A Parabola
How To Draw A Parabola - Find the coordinates of the vertex point. Create a function table and “build” two points on each side of the vertex. The function decreases through negative two, four and negative one, one. The function is a parabola that opens up. The taller you make it, the smoother your parabolic curve will be. Here when y = 0, we obtain two solutions. (or y = √x for just the top half) a little more generally: The equation of a tangent to the parabola y 2 = 4ax at the point of contact \((x_1, y_1)\) is \(yy_1 = 2a(x + x_1)\). Graphs of quadratic functions all have the same shape which we call parabola. all parabolas have shared characteristics. There are two pieces of information about the parabola that we can instantly get from this function. The graph is the function x squared. Find the focus for the equation y 2 =5x. The tangent is a line touching the parabola. X + 3 = 0 or x − 1 = 0 x = − 3 x = 1. Plot your points and construct your curve (parabola) now, let’s go ahead and apply these three steps to. Create a function table and “build” two points on each side of the vertex. Web to do this, set y = 0 and solve for x. The function decreases through negative two, four and negative one, one. Make sure they are evenly spaced and equal on both sides. Web let’s take a look at the first form of the parabola. The function decreases through negative two, four and negative one, one. Web to draw a parabola graph, we have to first find the vertex for the given equation. Set up a table with chosen values of x. On both sides of the angle. Web here we shall aim at understanding some of the important properties and terms related to a. X + 3 = 0 or x − 1 = 0 x = − 3 x = 1. The line drawn perpendicular to tangent and passing through the point of. Find the focus for the equation y 2 =5x. The tangent is a line touching the parabola. The taller you make it, the smoother your parabolic curve will be. (or y = √x for just the top half) a little more generally: Set up a table with chosen values of x. Here when y = 0, we obtain two solutions. The simplest equation for a parabola is y = x2. Turned on its side it becomes y2 = x. The graph is the function x squared. Add notches, dots, points, etc. Find the focus for the equation y 2 =5x. For example, they are all symmetric about a line that passes through their vertex. Here when y = 0, we obtain two solutions. (or y = √x for just the top half) a little more generally: Find the coordinates of the vertex point. Web let’s take a look at the first form of the parabola. Plot your points and construct your curve (parabola) now, let’s go ahead and apply these three steps to this first example: Make sure they are evenly spaced and. Web let’s take a look at the first form of the parabola. Graphs of quadratic functions all have the same shape which we call parabola. all parabolas have shared characteristics. The taller you make it, the smoother your parabolic curve will be. The equation of a tangent to the parabola y 2 = 4ax at the point of contact \((x_1,. The function is a parabola that opens up. On both sides of the angle. Find the focus for the equation y 2 =5x. The tangent is a line touching the parabola. Using a ruler, draw a line from the top left notch to the right of the bottom left botch. Create a function table and “build” two points on each side of the vertex. The simplest equation for a parabola is y = x2. F (x) = a(x −h)2 +k f ( x) = a ( x − h) 2 + k. Turned on its side it becomes y2 = x. The graph is the function x squared. First, if a a is positive then the parabola will open up and if a a is negative then the parabola will open down. Find the focus for the equation y 2 =5x. In this form the vertex is the point (h, k), and you don't need to do any math to find the vertex beyond interpreting the graph correctly. F (x) = a(x −h)2 +k f ( x) = a ( x − h) 2 + k. Find the coordinates of the vertex point. Find the coordinates of the vertex point. The taller you make it, the smoother your parabolic curve will be. There are two pieces of information about the parabola that we can instantly get from this function. Turned on its side it becomes y2 = x. The line drawn perpendicular to tangent and passing through the point of. Web to do this, set y = 0 and solve for x. Where a is the distance from the origin to the focus (and also from the origin to directrix) example: Graphs of quadratic functions all have the same shape which we call parabola. all parabolas have shared characteristics. Web here we shall aim at understanding some of the important properties and terms related to a parabola. The tangent is a line touching the parabola. Plot your points and construct your curve (parabola) now, let’s go ahead and apply these three steps to this first example: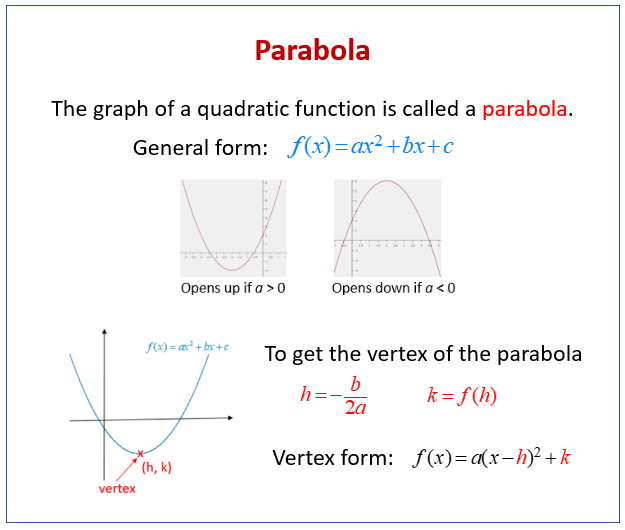
Graphing Parabolas (examples, videos, solutions, activities)

Come Disegnare una Parabola 13 Passaggi (Illustrato)

Parabolas2of8 How to draw a parabola given the focus and directrix

How to draw a parabola given its focus and its directrix YouTube

Easy Steps to Draw a PARABOLA by RECTANGLE METHOD Engg Curves Engg

How to Graph a Parabola in a Cartesian Coordinate System Owlcation

How to draw a parabola? GeoGebra

How To Write An Equation For A Parabola INFOLEARNERS
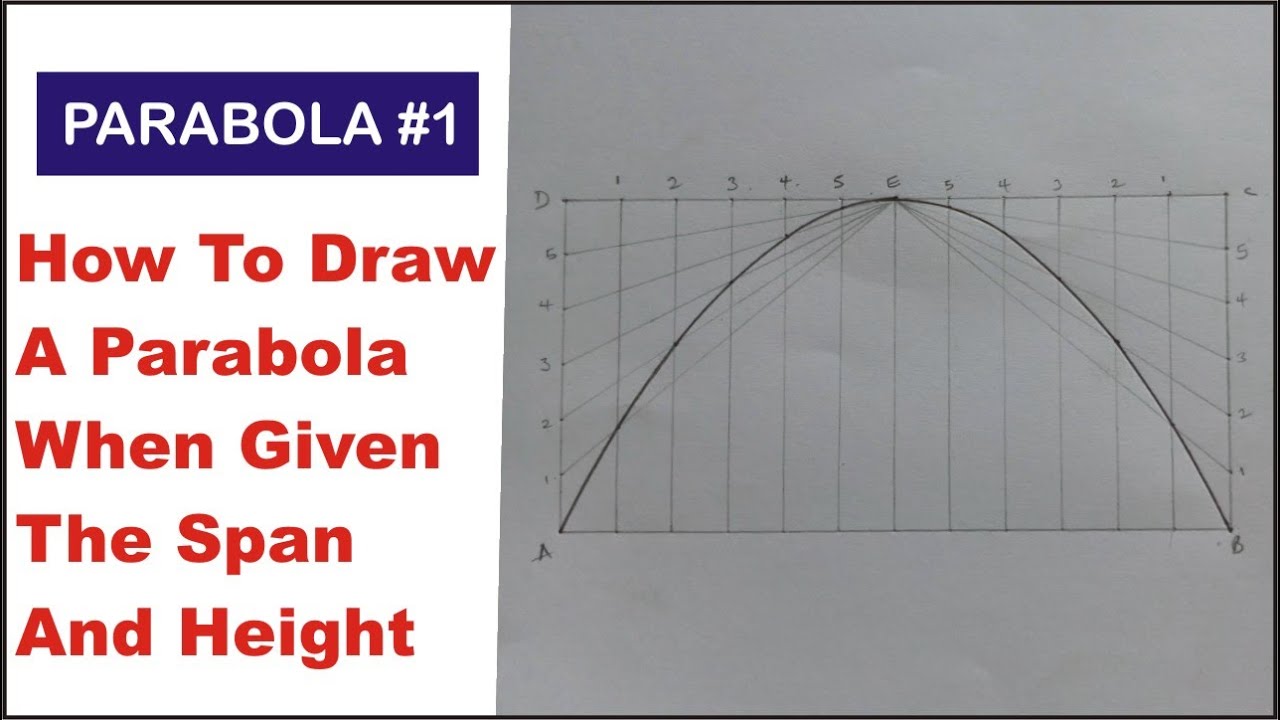
PARABOLA 1. How to Draw a PARABOLA when Given the Span and the Height

How to Draw Parabola By Tangent Method Engg Curves Engg Drawing
Using A Ruler, Draw A Line From The Top Left Notch To The Right Of The Bottom Left Botch.
The Simplest Equation For A Parabola Is Y = X2.
For Example, They Are All Symmetric About A Line That Passes Through Their Vertex.
The Equation Of A Tangent To The Parabola Y 2 = 4Ax At The Point Of Contact \((X_1, Y_1)\) Is \(Yy_1 = 2A(X + X_1)\).
Related Post: